
Menu

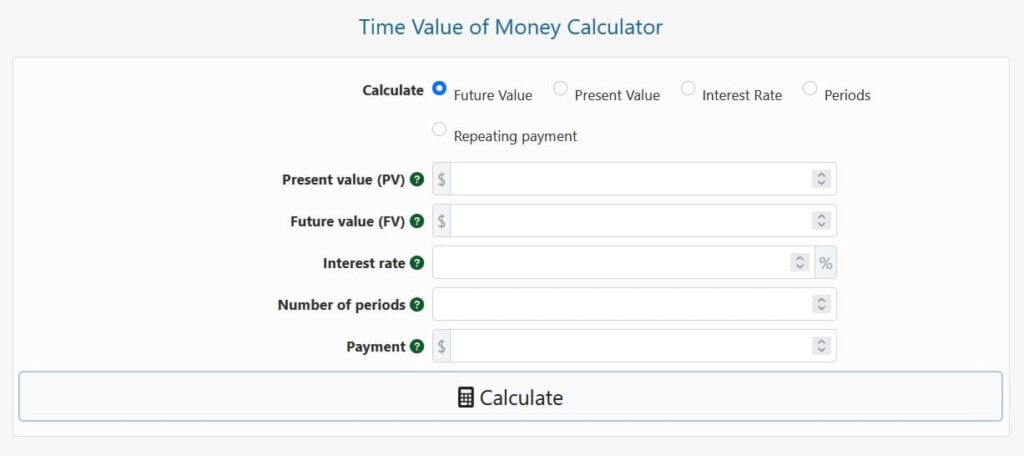
Our online calculator is a straightforward and easy-to-use tool for calculating numerous quantities linked to the time value of money, such as present value, future value, interest rate, and the number of payments required to repay a loan or increase the value of a deposit to a specific amount. Provide the remaining values and hit “Calculate” when you’ve decided what you want to compute for. If you’re not sure what to do, look at the question marks next to each field’s label.
If the figures reflect cash outflows, enter them with a minus in front of them as a general rule. If you’re depositing or investing $100, for example, enter -100. If you’re taking out a $100 loan, just type “100.” If you’re withdrawing money from an investment or deposit, write “-20,” and if you’re depositing $20 to cover the interest or principle on a loan/credit, enter “20.”
Note that the interest rate you enter in the calculator’s interest rate box must reflect the effective interest rate for the period you’re calculating. If the period is a year (for example, if the “Number of periods” option is “5” and the loan is for five years), you should enter the effective annualised interest rate. Instead, if the term is a month, enter the effective monthly interest rate.
The powerful concept of time value of money underscores the basic truth that humans have a preference for timing: given identical profits, they would choose to take them now rather than later. For example, if you have the option of receiving $10,000 now or in five years, you would select the latter. This is due to the possibility to use the money right away (nearly definite advantage) as well as the uncertainty of spending them in 5 years. Similarly, if you invest the funds or place them in a bank account, you can earn interest over the course of the five years, which you would not be able to do otherwise.
The existence of interest rates is due to time preference: they represent the “price” paid for using money for a specific period of time. It makes up for the depositor’s or lender’s lost opportunity cost. As a result, interest rates are low when the perceived opportunity cost is low and high when the perceived opportunity cost is high. Similarly, investors weigh the projected return (growth in the value of their capital) vs alternative investments when deciding whether to engage in a certain endeavour.
The present value (PV), future value (FV), value of individual payments in each compounding period (A), number of periods (n), and interest rate are all used to calculate the temporal value of money (TVM) (r).
To compute present value and future value without periodic payments, apply the following two formulas:

Periodical payments must be modified in the same way as the present and future values and added to the calculations above. Calculating the amount of the required periodic payment is a straightforward analytical transformation that is performed automatically by the TVM solver.
However, because there is no analytical answer, calculating the number of periods or the interest rate is not simple. As a result, several approximations must be made until a suitably precise value is found. This is something that our time value of money calculator can readily achieve for you.
With the help of our online TVM solver, making better wealth management decisions can become a little easier. The solver may calculate:
Furthermore, more sophisticated formulas can contain a rising rather than a set periodic payment (g), although this is not currently supported by the TVM calculator.
After 5 years, the total value is $11,041, but it would have been only $11,000 with simple interest. This may not appear to be a significant amount, but if the rate of return is higher or the compounding period is longer, the compounding effect can be significant.
© 2021 All rights reserved
Ask Your Query